Eco-evolutionary dynamics of living systems: Theory
Adaptive dynamics theory, which was co-developed by EEP researchers, has become widely used in studies interfacing ecological, evolutionary, and environmental changes.
- One of the intriguing features of adaptive dynamics theory is its capacity for predicting the endogenous diversification of evolutionary traits at so-called evolutionary branching points. The underlying bifurcations have now been clarified for multivariate traits [1][2][3].
- Extending earlier EEP work on adaptations in function-valued traits, new research synthesized two previously isolated approaches and enabled particularly efficient calculations [4].
- A new algebraic characterization of fitness functions implying evolutionary optimization, often encountered in the literature, enables a precise assessment of such models’ lack of robustness [5]. EEP also continued its work on understanding the spatial structure of ecosystems:
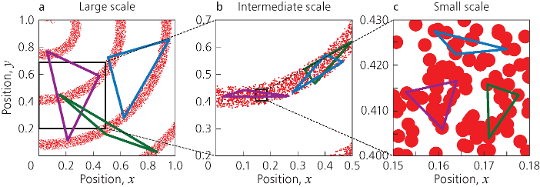
- Spatial moments of increasing order enable researchers to focus on the essentials of such structure: the first moment measures the density of individuals, the second moment the density of pairs of individuals at a given distance, the third moment the density of triplets of individuals in a triangular configuration, and so on. A new EEP study has now elucidated for the first time how to extract, interpret, and use the information captured by third spatial moments [6] (Figure 1).
Figure 1. Illustration of how the prevalence of triangular configurations in a point pattern changes with their spatial scale [6].
References
[1] Della Rossa F, Dercole F & Landi P (2015). The branching bifurcation of adaptive dynamics. International Journal of Bifurcation and Chaos 25: 1540001.
[2] Dercole F, Della Rossa F & Landi P (2016). The transition between evolutionary branching and evolutionary stability. Nature Scientific Reports, in press.
[3] Geritz SAH, Metz JAJ & Rueffler C (2015). Mutual invadability near evolutionarily singular strategies for multivariate traits, with special reference to the strongly convergence stable case. Journal of Mathematical Biology, in press. doi:10.1007/s00285-015-0944-6.
[4] Metz JAJ, Stanková K & Johansson J (2015). The adaptive dynamics of life histories: From fitness-returns to selection gradients and Pontryagin’s maximum principle. Journal of Mathematical Biology Online First: doi 10.1007/s00285-015-0938-4.
[5] Metz JAJ & Geritz SAH. Frequency dependence 3.0: An attempt at codifying the evolutionary ecology perspective. Journal of Mathematical Biology 72: 1011–1037.
[6] Kaito C, Dieckmann U, Sasaki A & Takasu F (2015). Beyond pairs: Definition and interpretation of third-order structure in spatial point patterns. Journal of Theoretical Biology 372: 22–38.

CONTACT DETAILS
Principal Research Scholar Exploratory Modeling of Human-natural Systems Research Group - Advancing Systems Analysis Program
Principal Research Scholar Systemic Risk and Resilience Research Group - Advancing Systems Analysis Program
Principal Research Scholar Cooperation and Transformative Governance Research Group - Advancing Systems Analysis Program
Research program